AFAIK, they are used as relays.

From https://en.wikipedia.org/wiki/1-bit_computing#1-bit

Computers and microcomputers may also be used, but they tend to overcomplicate the task and often require highly trained personnel to develop and maintain the system. A simpler device, designed to operate on inputs and outputs one-at-a-time and configured to resemble a relay system, was introduced. These devices became known to the controls industry as programmable logic controllers (PLC).

See also the playlist linked in the other comment with more explanations:
1-Bit Breadboard Computer - Usagi Electric (YouTube)

For those curious about 1-bit computers, see Usagi Electric’s playlist:

• 1-Bit Breadboard Computer - Usagi Electric (YouTube)

Source: Sartrean Freedom - Existential Comics

RSS Feed: https://www.existentialcomics.com/rss.xml

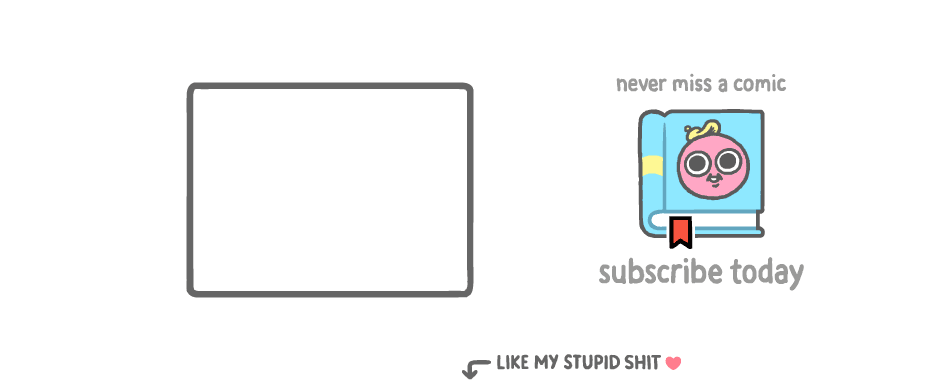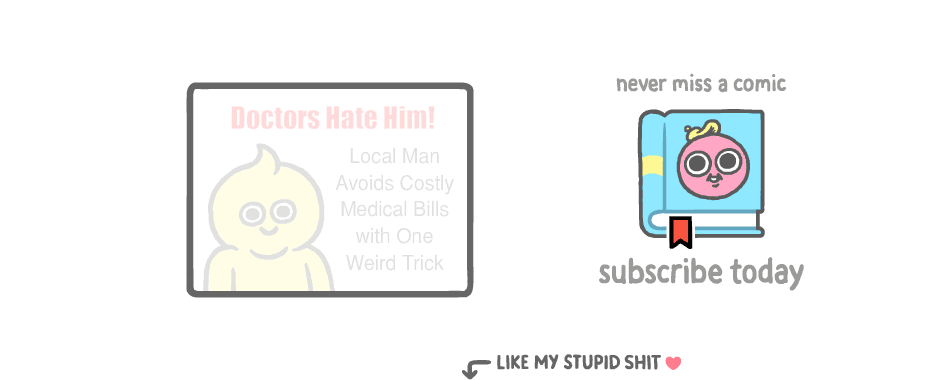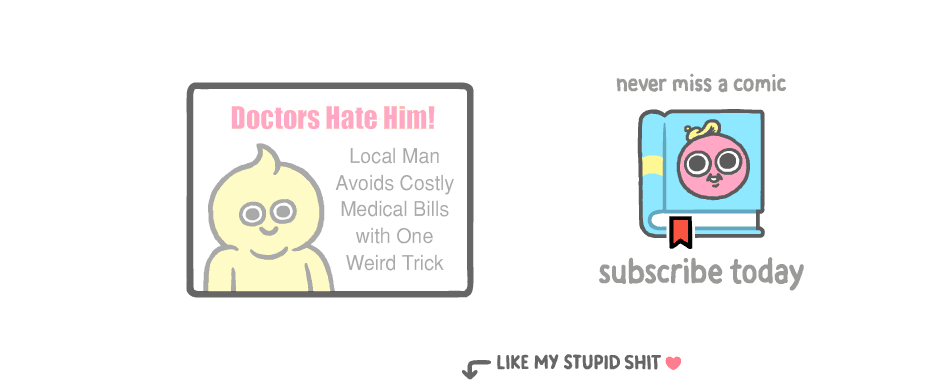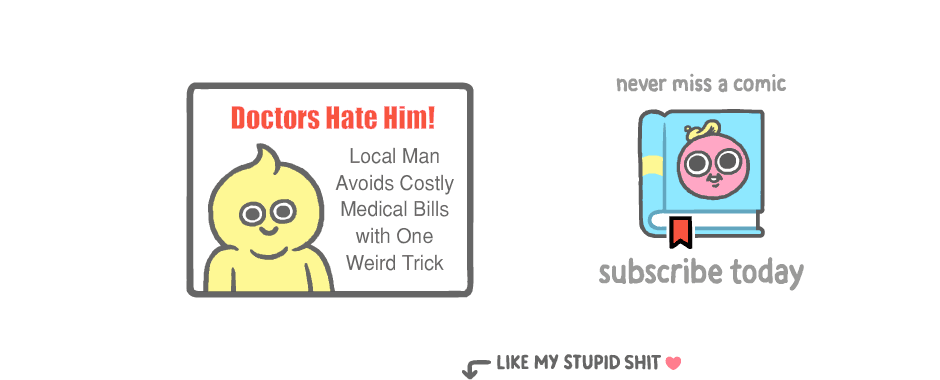
Source: Fossil Fools #135 - Minim (Calligraphy)

I don’t see an RSS Feed on their site, so here it is the RSS Feed for u/fossilfoolscomic’s submissions to r/comics:
https://www.reddit.com/r/comics/search/.rss?q=author:“fossilfoolscomic”&include_over_18=on&restrict_sr=on&t=all&sort=new

Not the first time someone says it fails.

But I cannot get it to fail, it works for me.
You can try the RSS button on their Tapas profile: https://tapas.io/series/Doodle-Time/info

Source: Read Sarah’s Scribbles :: Poltergeist | Tapas Comics

RSS Feed: https://tapas.io/rss/series/2007

!fairphone@lemmy.ml

Source: Sunny Street by Max G and Sandy B for April 07, 2014 - GoComics

RSS Feed: https://rsshub.app/gocomics/sunny-street

I think you’re confusing “arbitrarily large” with “infinitely large”. See Wikipedia Arbitrarily large vs. (…) infinitely large

Furthermore, “arbitrarily large” also does not mean “infinitely large”. For example, although prime numbers can be arbitrarily large, an infinitely large prime number does not exist—since all prime numbers (as well as all other integers) are finite.

For integers I disagree (but I’m not a mathematician). The set of integers with infinite digits is the empty set, so AFAIK, it has probability 0.

Doesn’t it depends on whether we are talking about real or integer numbers?

EDIT: I think it also works with p-adic numbers.

I also think that’s correct… if we are talking about real numbers.

People are probably thinking about integers. I’m not sure about OP.

EDIT: I think it also works with p-adic numbers.

That rings a bell

I read it as a critique of human tribalism. As if aliens came and helped those they encountered, their enemies would see it as an offence to them.

AFAIK, Port Sherry’s comics are usually not part of larger series.

From a similar post:

I suggest Rhizoverse, based on Deleuze’s and Guattari’s Rhizome philosophical concept.

But it’s probably too pretentious :)

Sources:

• Tapas.io (with secret panels): Shopping - Tapas.io RSS Feed

• Tumblr: Shopping - Tumblr RSS Feed

• Reddit: Shopping - r/mrlovenstein RSS Feed

Source: I asked Microsoft’s image creator to create “Godzilla in a 512k Mac game” and while this is not what I asked for, I can’t help but be impressed. - lemm.ee

Source: xkcd: Old Game Worlds

Hover text:

Ok, how many coins for a cinnamon roll?

Source: StormyGail Art (Twitter)

Nitter link

Nitter RSS Feed: https://nitter.cz/stormygailart/rss

Web: https://stormygailart.com/